The pH scale measures the acidity or alkalinity of a solution. The pH of a solution can be measured using a pH probe, or estimated using universal indicator and a colour chart.
The pH scale measures the acidity of an aqueous solution. Its values ranges from 0 to 14 (7 being the neutral value of pure water at 25ºC and 1 atm).
Lower pH value are acidic, higher values are basic.
pH can be measured with a pH meter, or with pH paper (paper containing a mixture of indicators to cause a continuous color change).
pH is a measure of the dissociation of an acid or base, and also of the concentration of that acid / base (actually its related to the concentration of H3O+ ions).
If we have two solutions with their pH values, the lower one will be more acidic and the higher one will be more basic (though they could both still be basic/acidic with respect to water -- pH 7).
Relationship between pH and acid concentration
A change of 1 in the pH scale represents a 10 times change in the acidity or basicity of the solution (because it's a log scale).
pH = - log10 [H+]
The concept of pH comes from a consideration that acidity is due to the presence of H+ ions in the water. These H+ ions come from the reversible breakdown of the water H2O molecules.
H2O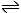 
H+ + OH-
H+ + OH-
It may be seen that the breakdown of one molecule of water will produce 1 of each of the resulting ions.
We could therefore measure acidity by simply measuring the concentration of hydrogen ions in the sample. This would, however, lead to very small and inconvenient numbers as the concentration in pure water at 25ºC is actually 1 x 10-7 moles dm-3
An attempt is made to produce numbers that are more easily handled using logarithms (i.e. expressing the number as the power to which 10 has to be raised to achieve the number - this is a common mathematical artifice)
log 1 x 10-7 = -7
This gives very small numbers a negative value and so a simple change of sign is performed making it positive. Thus the expression for potential hydrogen (pH) is:
pH = - log10[H+]
Low pH value are acidic, higher values are basic.
pH 1 represents strong acid, pH 7 is neutral, pH 14 represents strong base
Example:
Calculate the pH of a 0.01 moles dm-3 solution of a strong acid HCl.
As the acid only contains one hydrogen atom it will only produce one hydrogen ion per molecule when dissolved in water.
Therefore the H+ concentration is = 0.01 moles dm-3
log 0.01 = -2
pH = 2
|
The pH range
At 25ºC the equilibrium H2O 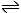H+ + OH- gives a value for both the H+ and OH- ions concentrations as 1 x 10-7
H+ + OH- gives a value for both the H+ and OH- ions concentrations as 1 x 10-7
According to the equilibrium law, Kc (the equilibrium constant) must remain the same providing the conditions remain the constant.
therefore:
Kc = [H+] x [OH-] /[H2O]
As the water concentration is very large in comparison to the other concentrations, slight changes in H+ and OH- do not affect it and so we may remove it from the equation, defining a new constant Kw (called the ionic product of water).
Kw will always be equal to 1 x 10-14 at 25ºC and consequently any increase in [H+] must be accompanied by a corresponding decrease in [OH-] to maintain the constant value of Kw.
The other consequence of this is that the pH range becomes 0 to 14 under the usual concentration conditions of the laboratory.
pH 1 represents strong acid, pH 14 represents strong base
Example : Calculate the pH of a 0.01 moles dm-3 solution of a strong base NaOH
As the base only contains one OH group it will only produce one hydroxide ion per molecule when dissolved in water.
Therefore the OH- concentration is = 0,01 moles dm-3
As the ionic product of water at 25ºC [H+] x [OH-] = 1 x 10-14
Then [H+] = 1 x 10-12
log 1 x 10-12 = -12
pH = 12
|
No comments:
Post a Comment